How to create a frequency table, Class Interval
Presentation
of data in frequency distribution is one of the first steps that is usually
done in analyzing a data. Interpretation of data can usually be made easier if
the data is organized and simplified first into a table. One of them is with
frequency table. Frequency distribution is a table, where data is grouped into
several numerical intervals called class intervals. The form of this table is
very simple because it only presents the number of observations or frequencies
in each class interval.
Frequency
table is a table that shows the distribution or distribution of data
frequencies that we have, which are composed of frequencies for each class or
category that have been set. The frequency of each class / category shows the
number of observations in the class category concerned.
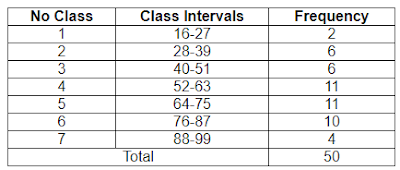
To
clarify the above description, we consider the frequency table as shown by the
following table,
By
studying the frequency table above, at least the description of the amount of
capital owned by 50 companies in New York can be known precisely, at least we
know immediately the distribution of company categories based on the amount of
capital owned by this frequency distribution, especially the distribution of
theoretical frequencies, plays a role important in statistical analyzes and
conclusions.
The
process of compiling data into a frequency distribution is very simple but
quite tedious and time-consuming if done manually. The stages needed in the
preparation of frequency distribution with class which is an interval /
interval is done in 4 steps namely;
Determination of the number of class intervals
The
number of class intervals, whether later making these tables using computer
assistance or not, we ourselves must determine. The number of class intervals
depends on the intent and purpose we make the frequency table and the number of
observations of the variables we have.
Observations
that are not too many certainly do not require a lot of class intervals, on the
contrary large observations require quite a lot of classes. However, so that
the number of class intervals obtained is easier to adjust, use the following
formula:
k = 1 + 3.3 log (n2/100)
The
formula is a modification of the Sturger formula, k = 1 + 3.3 log (n).
The
number of class intervals is also very dependent on the number of observations
in the data. The greater the amount of data, the more number of classes
required. However, it is usually recommended that the number of class intervals
range from 5 to 15 classes for a number of n between 50 and 1000. If the number
of class intervals used is too small, then we do not have much additional
information obtained from the grouping. Likewise, if the number of class
intervals is too much, then grouping data into class intervals will not provide
maximum benefit.
Determination of Class Intervals
The
interval in class or width depends on the number of classes chosen and the
range of data. One thing to note, not to complicate in interpreting the
frequency table, it is recommended that all class intervals have the same class
interval or class width.
Determination
of the width of the class interval is done by first determining the range
(Range) of the data, which is the difference between the highest observation
data with the lowest observation data, then dividing it by the number of
intervals desired.
R = Xb - Xk
Note: R = range
Xb = largest data
Xk = smallest data
Next, the intervals in the class we represent with I are determined by the formula:
I =
R / k
Determination of Class Interval Limits.
The
boundaries between class intervals must be clearly defined and not overlap so
that the values of observations can be grouped into each class, except for
open class intervals. Each class must have a lower limit and upper limit of the
class. The lower limit of the first class interval is usually the minimum value
of the data. While the upper limit of the last class interval is determined
such that the maximum value of the data lies in the last class interval.
A
class is called an open class interval if the class has no lower or upper
limits. Open class intervals are usually used if the data analyzed has very
large diversity and most observations are concentrated in a relatively small
range.
Determination of frequency for each class interval.
Determination
of the frequency for each class we will discuss here is if we do it manually
without the help of a computer.
There
are two events that can be used in determining the frequency of each class:
First,
we rearrange the data in sequence from the smallest to the largest or vice
versa. Next we count the number of observations including the first, second,
and so on class intervals up to the last class interval.
Second,
Enter the data one by one starting from the first observation into the class in
accordance with marking the class.
Example
of Making a Frequency Table
The
data of Mathematics Insurance student subject X is known in the range of 0-100,
with the highest value obtained is 98 and the lowest is 34. Where the data is
as follows:
79,
49, 88, 74, 81, 98, 87, 80, 80, 35, 48, 70, 91, 93, 87, 75, 68, 60, 76, 38, 56,
43, 74, 86, 90, 91, 83, 51, 65, 59, 71, 63, 63, 93, 88, 80, 68, 79, 75, 55, 69,
59, 73, 63, 79, 44, 89, 34, 85, 95.
From the data set above to create a Frequency Table, the steps that must be taken are:
Determination of the number of class intervals
To determine the number of classes of values that must be known in advance is the number of collected values is 50. If n = 50 is substituted into the formula to be,
Number of classes = 1 + (3.3) log 50 = 1 + 6.6 ≈ 7
Determination of Class Intervals
As is known the greatest value obtained from the Mathematics Insurance courses is 98 and the smallest is 34. So,
R = Xb
- Xk = 98 – 34 = 64
I
=
R / k = 64 / 6.6 = 9.7 ≈ 10.
The number 10 is the width of the class that must be made, for example, from grades 34 to (34 + 10) (counting starts from 34, 35, 36, 37 ..., 34).
From the calculation results above, the table can be presented as below,
Class
|
Number of Students (fi)
|
Frequency(%)
|
34
– 43
|
4
|
8
= (4/50) x 100
|
44
– 53
|
4
|
8
|
54
– 63
|
8
|
16
|
64
– 73
|
7
|
14
|
74
– 83
|
13
|
26
|
84
– 93
|
12
|
24
|
94
– 103
|
2
|
4
|
Total
|
50
|
100
|
Note:
To get the number of students and the frequency it is better to arrange the
data in advance can be manually or using Excel software.
SUBSCRIBE TO OUR NEWSLETTER
0 Response to "How to create a frequency table, Class Interval"
Post a Comment